To calculate result you have to disable your ad blocker first.
Washer Method Calculator
To calculate volume of the solid revolution, enter f(x), g(x), and upper & lower limit into the required input fields and click the calculate button using washer calculator
Washer Method Calculator
Washer method calculator finds the volume of the solid revolution to cover the sold with a hole by using a definite integral. This washer calculator finds the definite integral of the sum of two squared functions (f(x)2 + g(x)2) and multiplies it by π (pi).
What is the washer method?
In geometry, a washer method is used to find the volume of different kinds of solid shapes such as a round shape with a hole in the center. The shapes are obtained by rotating two functions around the x-axis and y-axis.
Formula of washer method
The formula for the washer method is:
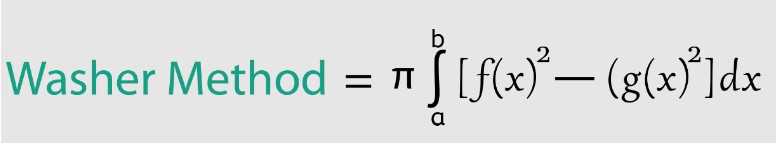
How to calculate the problems of the washer method?
The washer method calculator above can solve the problems in a couple of seconds. Below is a solved example to calculate the washer method manually.
Example
Find the volume of the solid revolution if the functions are f(x) = x2 + 4 & g(x) = 4x + 1 in the interval of [2, 4].
Solution
Step 1: Take the given information.
f(x) = x2 + 4
g(x) = 4x + 1
upper limit = 4
lower limit = 2
Step 2: Take the formula of the washer method.
Washer method = π\(\int _a^b\:\)[f(x)2 + g(x)2] dx
Step 3: Calculate the sum of squares of the functions
f(x)2 = (x2 + 4)2 = x4 + 8x2 + 16
g(x)2 = (4x + 1)2 = 16x2 + 8x + 1
f(x)2 + g(x)2 = x4 + 8x2 + 16 + 16x2 + 8x + 1
f(x)2 + g(x)2 = x4 + 24x2 + 8x + 17
Step 4: Find the definite integral of the above function with respect to “x”.
\(\int _2^4\:\)[f(x)2 + g(x)2] dx = \(\int _2^4\:\)[x4 + 24x2 + 8x + 17] dx
= \(\int _2^4\:\)[x4] dx + \(\int _2^4\:\)[24x2] dx + \(\int _2^4\:\)[8x] dx + \(\int _2^4\:\)[17] dx
= [x4+1] dx + \(\int _2^4\:\)[24x2] dx + \(\int _2^4\:\)[8x] dx + \(\int _2^4\:\)[17] dx
\(=\left[\frac{x^{4+1}}{4+1}\right]^4_2+24\left[\frac{x^{3+1}}{3+1}\right]^4_2+\:8\left[\frac{x^{1+1}}{1+1}\right]^4_2\:+\:17\left[x\right]^4_2\)
\( =\left[\frac{x^5}{5}\right]^4_2+24\left[\frac{x^4}{4}\right]^4_2+\:8\left[\frac{x^2}{2}\right]^4_2\:+\:17\left[x\right]^4_2\)
\( =\frac{1}{5}\left[x^5\right]^4_2+\frac{24}{4}\left[x^4\right]^4_2+\:\frac{8}{2}\left[x^2\right]^4_2+17\left[x\right]^4_2\)
\( =\frac{1}{5}\left[4^5-2^5\right]+6\left[4^4-2^4\right]+4\left[4^2-2^2\right]+17\left[4-2\right]\)
\( =\frac{1}{5}\left[1024-32\right]+6\left[256-16\right]+4\left[16-4\right]+17\left[4-2\right]\)
\( =\frac{1}{5}\left[992\right]+6\left[240\right]+4\left[12\right]+17\left[2\right]\)
\( =198.4+1440+48+34\)
\(\int _2^4\:\left[x^4+24x^2+8x\:+17\right]\:dx=1720.4\)
Step 5: Substitute the values in the formula.
Washer method = π\(\int _2^4\:\)[x4 + 24x2 + 8x + 17] dx
Washer method = π(1720.4)
Put π = 3.14
Washer method = (3.14)(1720.4) = 5402.056

